 |
| Image 1 |
The one thing about math I have always taken solace in is
the fact that there is one answer. That one answer poses a challenge that I
feel I have to meet. It is just like reading a book, doing a puzzle or even
watching a movie I need to see it all come to one concrete end. Conversely to
these thoughts, I have also learned that math is not always straight forward,
it can be messy and complicated with multiple answers or there are those questions
that remain unanswered. Of course I still love the straight forward questions
but as I continue my work in mathematics, now through a teaching lens, I
realize that the real learning took place while working on the "messy"
questions. In order for students to work on these types of questions teachers
need to develop confident, independent and critical thinking in their students.
One way to gradually develop these skills is to implement open ended questions
in a lesson plan. I can tell you that at first trying to use open ended
questions in math is not as easy as english for example, but with practice it
becomes second nature. In my teaching mathematics class this week we discussed some
common and effective examples of open questions. Questions that leave a lot of
room for student interpretation are comparison questions; asking students how a
figure or an equation compares to another. Another key point we discussed was
how teachers should provide enough information to form a problem but not too
much information to completely restrict the question. Using words such as
"approximately", "minimum" or "maximum" can open
the question to multiple solutions. Below are two examples of open questions
where the approach was providing the answer and having the students work
backward to formulate the questions.
 |
| Image 2 |
 |
| Image 3 |
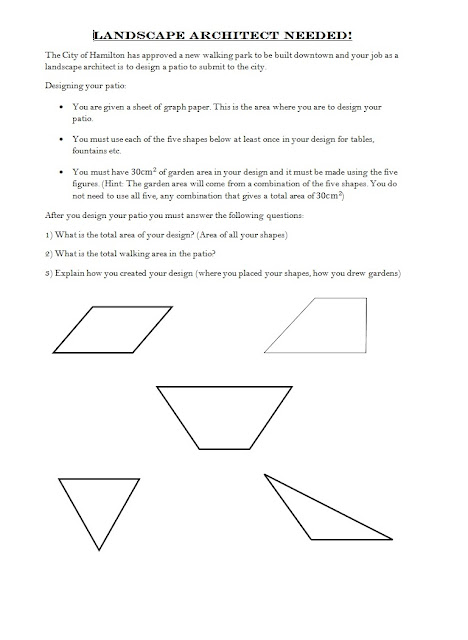
In one
of my placements during my undergrad, I taught the unit on area and perimeter to
the grade 7 class. One assignment I gave to the class was similar to one of the
unit problems from the textbook however I tweaked it making it more of an open
ended problem. The students were required to create a patio design under some restrictions
including using only the five specific shapes. (Prior to completing the problem
I had marked out the five shapes on the floor using tape and the students
calculated the area by measuring the floor tiles.) In the end each student had
submitted a completely unique patio design. As long as the design included the five
shapes and met the minimum area requirements then each of the designs were 100%
correct. I think this assignment was a good balance between open ended and
closed questions. After asking the students to formulate their own solutions to
the design, I then asked all of them the same closed questions which they had
to answer in relation to their own individual design area. I believe problems such
as these are beneficial to all students because at the very least everyone can
make an attempt and as I mentioned before this is a step towards building their
confidence when dealing with math.
 |
| Patio Problem |
Resources
Image 1 Retrieved from: https://s-media-cache ak0.pinimg.com/564x/11/0b/93/110b9301f2edcbad7f7c7dc959dcd19f.jpg
Image 2 Retrieved from: https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEjKbl0cmVgE7Pu7Rh6EMqmxtk4gk1rzRcyppG2o7h1UvTjFA-enDAPj_7cd8dLWN66ebNiFOvJaiyfjHCrjWtkGN64BfrPz07HWETAK1yNxzyMiwZwhzHnbvpeZ2yzYDxSM-UPgcot888r6/s1600/Maths_Challenge_2.png
Image 3 Retrieved from: https://s-media-cache-ak0.pinimg.com/564x/07/73/df/0773df99e143cf484de58669a140ff51.jpg
Hi Stefanie,
ReplyDeleteI love your idea of creating a patio design using five shapes. The creativity and innovative thinking involved in creating the patio allows students to have fun while using their critical and logical thinking. I have not considered using an open-ended technique alongside a closed, but the way you organized that assignment was quite fascinating. I agree with your point that offering problems such as your patio perimeter one, will build a students confidence in math. The formulation of a question is key, and taking away the restrictions of traditional math instruction will definitely encourage a more stress-free learning environment.